CSTR

exd2
Model of a continuous stirred tank reactor (CSTR).


Variables and parameters:

Da: Damköhler number (bifurcation parameter: 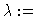$\lambda:=$Da)

y1 , y2: describe the material and energy balances

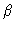$\beta$: the heat transfer coefficient 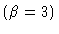$(\beta=3)$

B: the rise in adiabatic temperature (B=16.2)


CSTR model equations:

\begin{displaymath}\dot y_1 &= - y_1 + \hbox{Da}(1-y_1 ) \exp (y_2)\cr\end{displaymath}


\begin{displaymath}\dot y_2 &= - y_2 + B\cdot\hbox{Da}(1-y_1 ) \exp (y_2 ) - \beta y_2 \cr\end{displaymath}




Comments

Figure 1
Branching diagram y1(0) versus Da. One branch of stationary solutions, and two branches of periodic orbits branching off at two Hopf bifurcations.

Figure 2
y1(t) simulation for 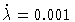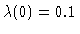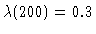$\dot \lambda=0.001$, $\lambda(0)=0.1$, $\lambda(200)=0.3$ with initial values y1(0)=0.1644, y2(0)=0.6658

Figure 3
y1(t) simulation for 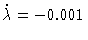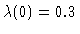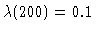$\dot \lambda=-0.001$, $\lambda(0)=0.3$, $\lambda(200)=0.1$ with initial values y1(0)=0.9279, y2(0)=3.76

Postscript-Files for better printing results:

This Example
Figure 1
Figure 2
Figure 3